|
Emotions and Reidentification
Section revised and extended October,
2000.
The basic condition for reidentification, defined in
proposition (67), makes no reference to emotional
states that are observed while events confirm or disconfirm people's situational
identities. Such a formulation is useful because in many instances emotional
states are unknown, poorly appraised, or purposely discounted. In other cases,
though, such a formulation is too simplistic.
[W]hen we are present at the events that stimulate reidentifications or when
we observe a person narrating his or her own behavior, we have access to the
expressive signaling system that our species has evolved, and we obtain
information about what emotions the person feels as a result of the events.
... Reassessment of the person's character must take account of the emotion
displayed because the expressive behavior may cue us that the other feels disconfirmed
by events rather than confirmed. (Heise, 1989, p. 14)
This section delineates two approaches to incorporating information on
emotions into reidentification processes. The first presumes that the target
individual is in a particular mood while experiencing an event, and an
observer tries to factor that mood into an inference about the identity that the
target individual must have. This formulation was introduced by Heise (1989). In
the second approach, an observer tries to infer the identity of the target
individual from seeing the emotion that a particular event triggers in the
target individual. This formulation is presented in the 2000 revision of this
web document for the first time.
Inferences From Mood
A mood is an emotional predilection being maintained
as an aspect of one's identity in a particular situation. The emotion defining
the mood amalgamates with one's role identity, generating the fundamental self
to be maintained in the situation. Equation (62c)
provides the relevant formulation of the amalgamation process for present
purposes:
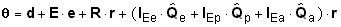 (75)
(75)
q is the amalgamate EPA profile, e
is the EPA profile for the emotion defining the individual's mood, and r
is the EPA profile for the individual's role identity. Vector d provides
equation constants, and 3x3 matricies E and R consist of
coefficients for weighting the first-order contributions of emotion and role
identity to the amalgamate. IEe is a 3x3 diagonal matrix with
the emotion evaluation in the diagonal cells, IEe is a 3x3
diagonal matrix with the emotion potency in the diagonal cells, and IEe
is a 3x3 diagonal matrix with the emotion activity in the diagonal cells, and
the Q matricies consist of coefficients for weighting the second-order
interactions of emotion and identity EPAs in generating the amalgamate.
The vector of EPA values for the amalgamate can be
substituted in equation (8) for the fundamental
profile of the actor in an event.
 (76)
(76)
or
 (77)
(77)
In this application, r' = [ e e
 p p
 a
], the fundamental EPA profile of the actor's identity, and a
], the fundamental EPA profile of the actor's identity, and 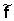 can be expressed as a function of the original f vector in equation (8)
can be expressed as a function of the original f vector in equation (8)
 (78)
(78)
by means of the following definitions:
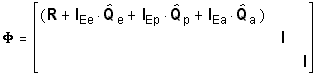 (79)
(79)
and
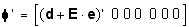 (80)
(80)
Equation (12), which
interprets unlikelihood as a sum of deflections, can be rewritten using 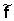 as follows:
as follows:
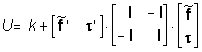 (81)
(81)
Substituting the expression for 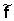 from
(86) gives from
(86) gives
[Section above revised and shortened October, 2000.]
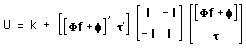 (90) (90)
or
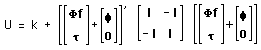 (91) (91)
where 0 is a vector of zeros whose order matches the order of t.
Multiplying out gives
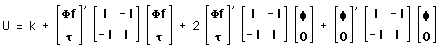 (92) (92)
or
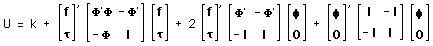 (93) (93)
At this point we use equation (15)
to express t in terms of transients existing
before the event:
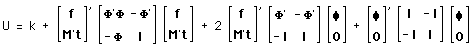 (94) (94)
or
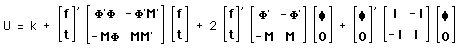 (95) (95)
This reduces to
 (96) (96)
Since the mood is treated as a given, phi is constant during the
reidentification, and the last term may be absorbed into the equation constant.
Doing this and rearranging terms gives
 (97) (97)
which has exactly the structure of (18), the
generalized equation for unlikeliness. Thus the solution for the optimal actor
is given by (47) with H in equation (29)
redefined as
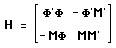 (98) (98)
and h in equation (30) as
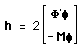 (99) (99)
Heise (1989) pointed out an interesting property of
the solution: the predicted evaluation of an actor is positive
if the actor's emotion is consistent with the impact of the actor's behavior
(e.g., feeling ashamed about an act with bad consequences or joyous about an act
with good consequences). The source of this effect is not obvious in the
equations above, but I will return to the issue when presenting an illustrative
analysis.
Inferences From Emotion
Instead of interpreting q in
equation (62c) as a fundamental self created from an amalgamation of mood and
role, we can interpret q as an individual's
transient state of being formed from the amalgamation of an emotion and role to
match the transient state of being created by an event.
Suppose that the individual is the actor in the event. Then q' =
[Ae Ap Aa]'.
Additionally from equation (15), [Ae Ap Aa]'
= t'MA, where M is the array of
impression-formation coefficients, and MA refers to the
portion that is used to predict impressions of an event's actor. Then q' =
t'MA, and from (62c)
 (99a)
(99a)
Interpret r as the individual's fundamental EPA profile
based on the individual's role identity. Then the question of what role identity
the individual must have in order to feel the given emotion as a result of the
given event is answered by solving for r.
 (99b)
(99b)
The event is represented in t, which is made up of the
pre-event transient EPAs for actor, behavior, and object, along with interaction
terms involving these transients.
   
URL:
www.indiana.edu/~socpsy/ACT/math/eq_6.html
Document: David
Heise, "Affect Control Theory's Mathematical Model, With a List
of Testable Hypotheses. A Working Paper for ACT Researchers."
February 7, 1992. Revised and posted on the World Wide Web, April 15,
1997.
|
| 